MPC Study Group: Oblivious Transfer Extension Part 2

In this week’s zoom meetup we looked at “More Efficient Oblivious Transfer Extensions with Security for Malicious Adversaries” by Asharov et al. (2015) [1]. We got stuck on the description of protocol 1 [1], which is the semi-honest secure OT extension protocol of Ishai et al. (2003) [2] (per notation from [3]) that we discussed last week, albeit with some optimizations. What we found was that the notation differed from what we discussed in last week’s session.

These are some personal study notes to clear up some of the confusion between the description from [1] and last week.

Differences and Similarities

The two protocols are shown in figures 1 and 2, in this section we refer to protocol 1 from [1] as fig. 1, and fig. 2 for [2, 3].

Figure 1: OT Extension, Protocol 1 from Asharov et al. (2015) [1]. Index $i$ is used for denoting columns, index $j$ for rows.

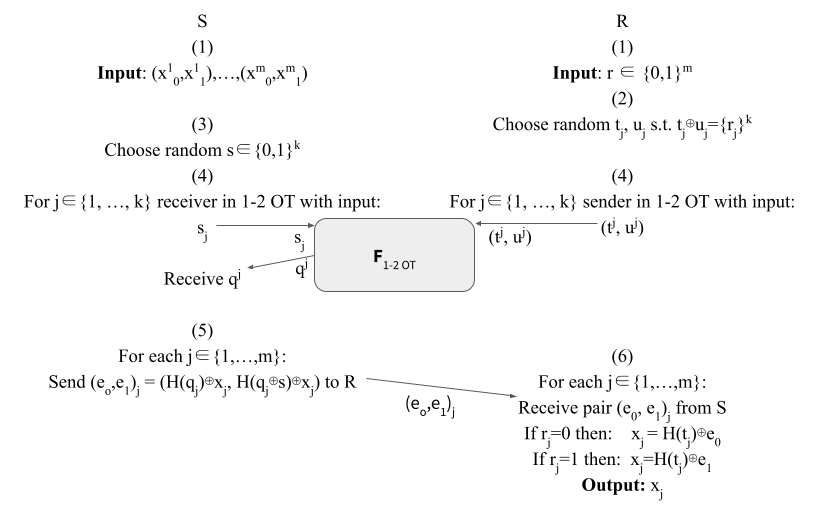
Figure 2: OT Extension, Ishai et al. (2003)[2], per notation from [3]. Index subscript $_i$ is used for denoting rows, index superscript $^i$ is used for denoting columns.

There are some immediately noticeable differences:

• Figure 1 [1] uses a pseudorandom generator (PRG): $G$, whereas figure 2 [2, 3] doesn’t.
• In figure 1 (1a), the receiver $R$ chooses random $k_i^0, k_i^1$ of length $k$, whereas in figure 2 in step (2) $R$ chooses $t_j, u_j$ of length $m$ randomly.
• Further, in fig. 1 the receiver $R$ transmits columns $k_i^0, k_i^1$ via the OT, whereas in fig. 2 $R$ transmits the columns $t^j, u^j$. This is somewhat confusing, as $k_i^0, k_i^1$ does not directly correspond to $t^j, u^j$. Further, in (2 a) of fig. 1 we define $t^j, u^j$ using $k_i^0, k_i^1$, and this definition differs from fig. 2.

The protocols may seem very different, but when we take a detailed look at $t^i, u^i$, $q^i$, etc. in both protocols we notice that the protocols are related.

• How are $T = t^0 | … | t^k$ and $U = u^0 | … | u^k$ related?
  • Fig. 1:
    • $t^i \oplus u^j = G(k_i^0) \oplus G(k_i^0) \oplus G(k_i^1) \oplus r = G(k_i^1) \oplus r$
  • Fig. 2:
    • $t_i \oplus u_i = \{r_i\}^k$, from which we can infer:
    • $t^i \oplus u^i =r$
  • We can see that $t^i \oplus u^i$ differ by $\oplus G(k_i^1)$ for fig. 1 and fig. 2.
• How are $q^i$ related?
  • Fig. 1:
    • $q^i = (\{s_i\}^k * u^i) \oplus G( k_i^{s_i})$ // as defined
    • $= (\{s_i\}^k * (G( k_i^0) \oplus G( k_i^1) \oplus r)) \oplus G( k_i^{s_i})$ // replace $u^i$.
    • If $s_i = 0$ then: $q^i = G( k_i^0)$.
    • If $s_i = 1$ then: $q^i = (G( k_i^0) \oplus G( k_i^1) \oplus r) \oplus G( k_i^1 ) = G( k_i^0) \oplus r$
    • And if we replace $G(k_i^0)$ with $t^i$ we get:
    • If $s_i = 0$ then: $q^i = t^i$.
    • If $s_i = 1$ then: $q^i = t^i \oplus r$.
  • Fig. 2:
    • If $s_j = 0$ then: $q^j = t^j$.
    • If $s_j = 1$ then: $q^j = u^j = t^j \oplus r$.
    • Another way of writing this is as: $q^i = (\{s_i\}^k * t^i) \oplus t^i$.
  • $q^j$ is the same for both fig. 1 and fig. 2.
• How are $q_j$ related?
  • $q_j$ is the same for both fig. 1 and fig. 2, this follows directly from previous step $q^i$.
  • Fig. 1 and fig. 2:
    • $q_j = (\{r_j\}^k * s) \oplus t_j$.

At this point we should be convinced that the protocols are related.

Correctness of Protocol 1

To better understand the notation of Asharov et al. (2015), let us reason why the output from the protocol is correct in fig. 1 (and leaving security for a later discussion).

To show the correctness of the output, it should suffice to show that for any $j$ the output is $x_j = x^{r_j}_j$. Let us try the case when $r_j = 0$ (case $r_j = 1$ is similar):

• In (2 e): $x_j = y_j^{r_j} \oplus H(j, t_j)$
• $x_j = y_j^0 \oplus H(j, t_j)$ // replacing $r_j$ with $0$
• $x_j = x_j^0 \oplus H(j, q_j) \oplus H(j, t_j)$ // replacing $y_j^0$
• $x_j = x_j^0 \oplus H(j, (\{r_j\}^k * s) \oplus t_j) \oplus H(j, t_j)$ // replacing $q_j = (\{r_j\}^k * s) \oplus t_j$
• $x_j = x_j^0 \oplus H(j, (\{r_j\}^k * s) \oplus t_j) \oplus H(j, t_j)$ // replacing $r_j$ with $0$, case assumption
• $x_j = x_j^0 \oplus H(j, (\{0\}^k * s) \oplus t_j) \oplus H(j, t_j)$
• $x_j = x_j^0 \oplus H(j, \oplus t_j) \oplus H(j, t_j)$
• $x_j = x_j^0$ // qed

Why is Protocol 1 Insecure Against Malicious Adversaries?

Protocol 1 is secure for a malicious sender and semi-honest receiver [1]. But it is not clear why it is insecure for a malicious receiver. Although it is mentioned in [1] that a malicious receiver $R$ may choose different $r$ when computing $u^i$ in step (2 a) fig. 1, it is not elaborated any further how such an attack could work. Luckily this attack is described in Ishai et al. (2003) [2]. What we present here is an adapted version of the attack for our notation. The malicious receiver changes the $j’$th bit of $r$ from 0 to 1, for the $i’$th computation of $u^i$. Together with the assumption that the receiver knows the of the $j’$th input-pair, the receiver can learn the $i’$th bit of $s$:

• Let us assume that the malicious receiver $R$ has knowledge of an input pair $(x_{j’}^0, x_{j’}^1)$ for a $j’ \in \{0, …, m\}$, and wlog $r_{j’} = 0$, row $j \in \{0, …, m\}$, column $i \in \{0, …, k\}$.
• Note that $R$ has knowledge of $t_i’$ from the protocol execution.
• In (2 a):
  • For all $i \neq i’: u^i = t^i \oplus G(k_i^1) \oplus r$
  • Note that $r$ is $0$ at index $j’$, i.e. $r_{j’} = 0$
  • For $i’: u^{i’} = t^{i’} \oplus G(k_{i’}^1) \oplus r’$, where $r’$ is $r$ but with a $1$ at index $j’$:
    • For all $j \neq j’: r’_j = r_j$
    • For $j’$: $r’_{j’} = 1$
• In (2 d):
  • Note that:
    • For all $j \neq j’: q_j = (\{r_j\}^k * s) \oplus t_j$
    • For $j’$:
      • $q_{j’} = ( \hat{r} * s) \oplus t_{j’}$
      • where $\hat{r} = [0, …, 0, 1, 0, …, 0]$ is 0 at all indices except at index $i’$ where $\hat{r}_{i’} = 1$.
      • $q_{j’} \oplus s = ( \hat{r} * s) \oplus t_{j’} \oplus s$
      • $= ( [0, …, 0, 1, 0, …, 0] * s) \oplus t_{j’} \oplus s$
      • $= [0, …, 0, s_{i’}, 0, …, 0] \oplus t_{j’} \oplus s$
      • $= [s_1, …, s_{i’-1}, 0, s_{i’+1}, …, s_{k}] \oplus t_{j’}$
  • If $x_{j’}^1 = y_{j’}^1 \oplus H(j’, t_{j’})$ then output $s_{j’} = 0$.
  • If $x_{j’}^1 = y_{j’}^1 \oplus H(j’, t_{j’} \oplus [0, …, 0, 1, 0, …, 0])$ then output $s_{j’} = 1$.
  • Note:
    • Where $[0, …, 0, 1, 0, …, 0]$ is the vector of zeroes except at index $j’$.
    • Note that the sender sets $y_{j’}^1 = x_{j’}^1 \oplus H(j’, q_{j’} \oplus s)$
    • Correctness argument:
      • If $s_{i’} = 0$ then:
        • $y_{j’}^1 \oplus H(j’, t_{j’})$
        • $= x_{j’}^1 \oplus H(j’, q_{j’} \oplus s) \oplus H(j’, t_{j’})$
        • $= x_{j’}^1 \oplus H(j’, [s_1, …, s_{i’-1}, 0, s_{i’+1}, …, s_{k}] \oplus t_{j’} \oplus s) \oplus H(j’, t_{j’})$ // replace $ q_{j’}$
        • $= x_{j’}^1 \oplus H(j’, [0, …, 0, s_{i’}, 0, …, 0] \oplus t_{j’} ) \oplus H(j’, t_{j’})$
        • $= x_{j’}^1 \oplus H(j’, [0, …, 0, 0, 0, …, 0] \oplus t_{j’} ) \oplus H(j’, t_{j’})$ // because $s_{i’} = 0$
        • $= x_{j’}^1 \oplus H(j’, \oplus t_{j’} ) \oplus H(j’, t_{j’})$
        • $= x_{j’}^1$ qed.
      • Similar argument for $s_{i’} = 1$

Follow-up

There were other questions that were unanswered: 1) why is it sufficient to ensure the consistency of $r$ for the malicious security model; 2) how does the malicious security protocol in [1] work.

References

• [1] Asharov, G., Lindell, Y., Schneider, T. and Zohner, M., 2015, April. More efficient oblivious transfer extensions with security for malicious adversaries. In Annual International Conference on the Theory and Applications of Cryptographic Techniques (pp. 673-701). Springer, Berlin, Heidelberg. URL
• [2] Ishai, Y., J. Kilian, K. Nissim, and E. Petrank. 2003. “Extending Oblivious Transfers Efficiently”. In:Advances in Cryptology – CRYPTO 2003. Ed.by D. Boneh. Vol. 2729.Lecture Notes in Computer Science. Springer,Heidelberg. 145–161.
• [3] Evans, David, Vladimir Kolesnikov, and Mike Rosulek. Section 3.7 “A pragmatic introduction to secure multi-party computation.” Foundations and Trends® in Privacy and Security 2.2-3 (2017). URL